Our new manuscript discussing Bargmann invariant as a unifying concept to study and measure weak values and Kirkwood-Dirac (KD) distributions is out now!
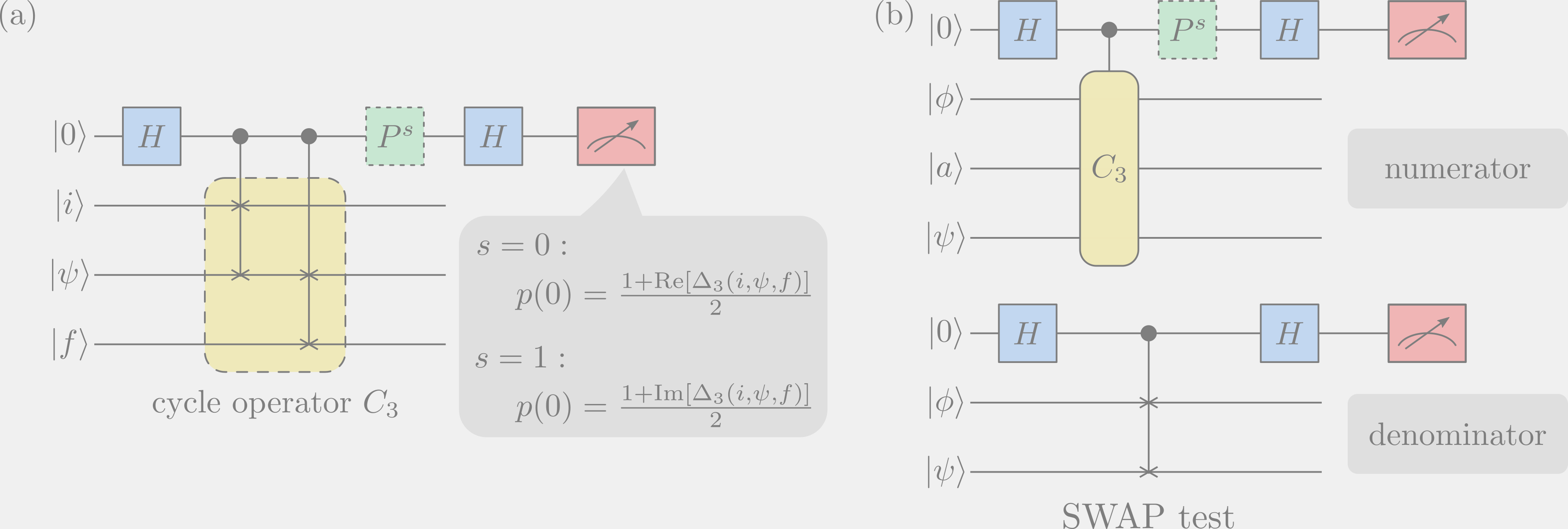
Bargmann invariants are unitary-invariant quantities that are associated with relational properties between a set of states and other fundamental concepts, such as geometric phases. Recently, it has been shown that they can be measured with simple circuits, called cycle tests.
In our new work, we show that Bargmann invariants are also a structure behind weak values and KD quasiprobability distributions. We also study the nonclassicality of the latter concepts from the perspective of Bargmann invariants since these invariants can be associated with the non-classical notion of set coherence.
You can click here to check the manuscript on the arXiv. This work was conducted in collaboration with Rafael Wagner, Zohar Schwartzman-Nowik, Amit Te’eni, Antonio Ruiz-Molero, Rui Soares Barbosa, Eliahu Cohen, and Ernesto F. Galvão.